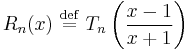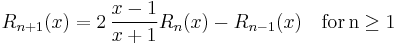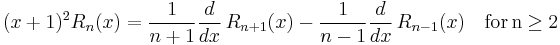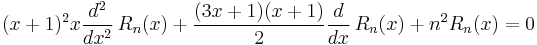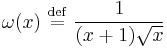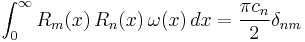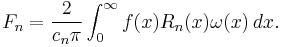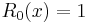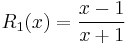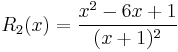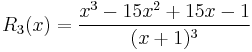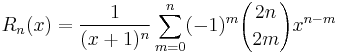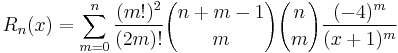Chebyshev rational functions
- This article is not about the Chebyshev rational functions used in the design of elliptic filters. For those functions, see Elliptic rational functions.
In mathematics, the Chebyshev rational functions are a sequence of functions which are both rational and orthogonal. They are named after Pafnuty Chebyshev. A rational Chebyshev function of degree n is defined as:
where 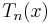 is a Chebyshev polynomial of the first kind.
is a Chebyshev polynomial of the first kind.
Contents |
Properties
Many properties can be derived from the properties of the Chebyshev polynomials of the first kind. Other properties are unique to the functions themselves.
Recursion
Differential equations
Orthogonality
Defining:
The orthogonality of the Chebyshev rational functions may be written:
where  equals 2 for n=0 and
equals 2 for n=0 and  equals 1 for
equals 1 for 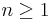 and
and 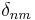 is the Kronecker delta function.
is the Kronecker delta function.
Expansion of an arbitrary function
For an arbitrary function  the orthogonality relationship can be used to expand
the orthogonality relationship can be used to expand 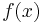 :
:
where
Particular values
Partial fraction expansion
References
- Ben-Yu, Guo; Jie, Shen; Zhong-Quing, Wang (2002). "Chebyshev rational spectral and pseudospectral methods on a semi-infinite interval" (PDF). Int. J. Numer. Meth. Engng 53: 65–84. doi:10.1002/nme.392. http://www.math.purdue.edu/~shen/pub/GSW_IJNME02.pdf. Retrieved 2006-07-25.